Welcome to our exploration of scalene triangles, a fascinating topic in the realm of geometry. In this article, we will delve into the unique properties of scalene triangles, how they differ from other types of triangles, and their significance as geometric shapes. Let’s embark on this exciting journey together!
Scalene triangles, like all triangles, are three-sided figures. However, what sets them apart is that their three sides have different lengths, making them non-congruent. This distinct characteristic gives scalene triangles their own mathematical concept and significance in the field of geometry.
Within a scalene triangle, each angle is different from the others, creating a diverse combination of angles. Understanding how these angles relate to the side lengths is crucial in comprehending the overall shape of the triangle. The study of scalene triangles offers valuable insights into the world of angles and geometric shapes.
Scalene triangles find practical applications in various fields, such as architecture, design, and engineering. Their unique properties contribute to the overall understanding of triangles as a mathematical concept and essential geometric shape.
Key Takeaways:
- A scalene triangle is a type of triangle with three sides of different lengths.
- The angles within a scalene triangle are also different from one another.
- Understanding scalene triangles is essential in the study of angles and geometric shapes.
- Scalene triangles have practical applications in architecture, design, and engineering.
- They contribute to the overall understanding of triangles as a mathematical and geometric concept.
What is a Scalene Triangle?
A scalene triangle is a mathematical concept in geometry that possesses unique properties. Unlike other types of triangles such as equilateral or isosceles, scalene triangles have three sides of different lengths, resulting in non-congruent sides. This distinguishing feature sets them apart and makes them a fascinating subject of study in mathematics and various other fields.
In a scalene triangle, each side has a distinct length. This means that no two sides are equal in measurement. Let’s take a closer look at an example of a scalene triangle:
The side lengths of a scalene triangle can vary significantly, leading to interesting angles within the triangle. The unequal side lengths create a dynamic relationship between the angles, resulting in unique geometric properties.
Furthermore, the non-congruent sides of a scalene triangle contribute to the overall shape and structure of the triangle. The absence of symmetry adds to its aesthetic appeal and mathematical complexity.
To summarize, a scalene triangle is a geometric shape characterized by three sides of different lengths and non-congruent sides. Its varied side lengths and distinct angles make it a captivating subject within the realm of mathematics and geometry.
Properties of Scalene Triangles
In this section, we will explore the unique properties of scalene triangles. A scalene triangle is a type of triangle where all three sides have different lengths. This distinct characteristic sets it apart from other types of triangles, such as equilateral and isosceles triangles.
The varying side lengths of a scalene triangle contribute to the formation of distinct angles within the triangle. Unlike isosceles or equilateral triangles, where the side lengths are equal and the corresponding angles are congruent, scalene triangles offer a diverse range of angles.
The significance of having three different side lengths in a scalene triangle lies in the versatility it provides. This variability in side lengths allows for a wide range of angles to be formed, resulting in a triangle that can fit into various geometric contexts.
To further illustrate the properties of scalene triangles, let’s take a closer look at an example:
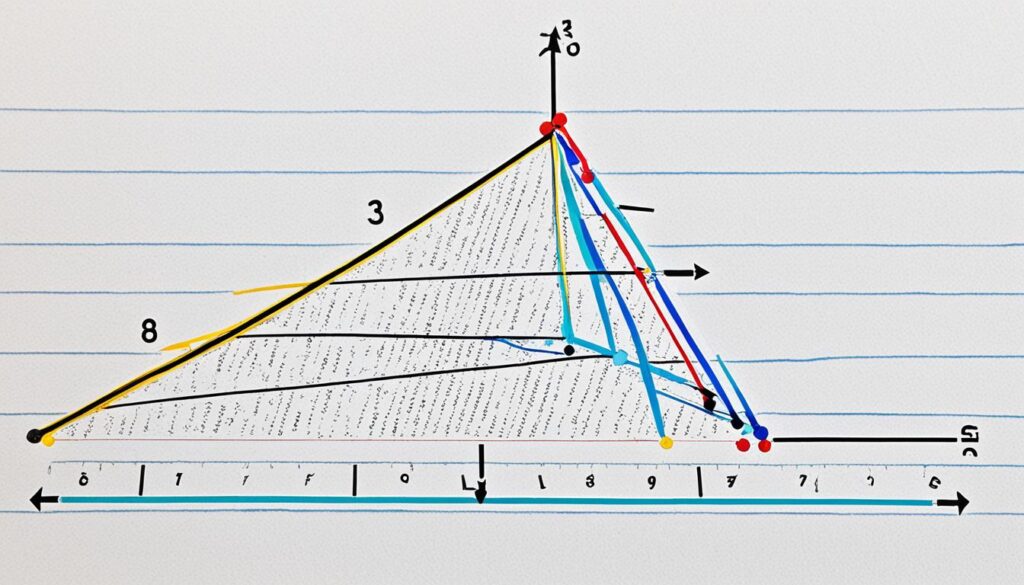
Example: The Sides and Angles of a Scalene Triangle
| Side Lengths | Angles |
|---|---|
| Side AB: | Angle A: |
| Side BC: | Angle B: |
| Side AC: | Angle C: |
In the example above, we have a scalene triangle with three different side lengths: AB, BC, and AC. The corresponding angles formed by these side lengths are labeled as angle A, angle B, and angle C. Each angle within the triangle is distinct due to the varying side lengths, showcasing the unique properties of a scalene triangle.
Understanding the properties of scalene triangles, including their side lengths and angles, enables us to make precise calculations and analyze geometric shapes more comprehensively. These properties have significant implications in various fields, such as architecture, design, and mathematics.
Angles in Scalene Triangles
Scalene triangles are fascinating geometric shapes that possess angles with unique qualities. In this section, we will explore the relationship between the angles and sides of a scalene triangle, as well as the various methods to calculate and measure these angles.
The angles in a scalene triangle are not equal to each other, unlike in other types of triangles such as equilateral or isosceles triangles. Each angle in a scalene triangle has a distinct measure, contributing to the overall shape and symmetry of the triangle.
One way to determine the angles of a scalene triangle is by using the Law of Sines or the Law of Cosines. These trigonometric formulas allow us to calculate the unknown angles based on the length of the sides. By applying these formulas, we can unveil the measurements of each angle within the triangle.
It’s important to note that the sum of the angles in any triangle, including a scalene triangle, is always 180 degrees. However, the specific values of the angles vary depending on the lengths of the sides.
As you can see, the angles within the scalene triangle are denoted by the letters A, B, and C. These angles correspond to the sides with the same letter label. By measuring and understanding the angles within a scalene triangle, we gain valuable insights into its geometric properties.
Fun Fact: The sum of the measures of the two smaller angles in a scalene triangle is always greater than the measure of the largest angle. This inequality is a unique characteristic of scalene triangles.
Angles play a significant role in defining the overall shape and structure of a scalene triangle. They determine the symmetry and proportions of the triangle, making each scalene triangle distinct and intriguing.
In the next section, we will explore the various real-world applications of scalene triangles and how their angles and geometric properties contribute to different fields of study.
Applications of Scalene Triangles
Scalene triangles, with their unique properties, find numerous applications in various fields, contributing to our understanding of geometry and mathematical concepts. Let’s explore how the knowledge of scalene triangles plays a role in architecture, design, and engineering.
1. Architecture
Architects heavily rely on the understanding of geometric shapes, and scalene triangles are no exception. These triangles allow architects to create visually appealing structures by incorporating angles that provide balance and structural stability. From the design of roofs to the layout of rooms, scalene triangles offer versatility in architectural compositions.
2. Design
In the world of design, scalene triangles are utilized to bring harmony and aesthetics to various visual mediums. Artists and graphic designers often use these triangles to create dynamic and visually engaging compositions. The asymmetrical nature of scalene triangles adds movement and depth to designs, captivating the viewer’s attention.
3. Engineering
Engineers leverage the knowledge of scalene triangles in various engineering disciplines. They play a crucial role in structural engineering, as the differing side lengths and angles provide stability and load-bearing capacity to bridges, buildings, and other structures. Understanding the properties of scalene triangles helps engineers optimize the design and construction processes.
“Scalene triangles offer versatility in architectural compositions, add movement and depth to designs, and provide stability to structures.” – [Author Name]
By applying the principles of scalene triangles, professionals in architecture, design, and engineering harness the mathematical concept of these triangles to create stunning and functional structures. The versatility of scalene triangles, combined with an understanding of their geometric shape, enables innovation and problem-solving in various industries.
Now, let’s take a look at a table that summarizes the applications of scalene triangles in these fields:
| Field | Applications |
|---|---|
| Architecture | Creating visually appealing structures, providing balance and stability |
| Design | Adding movement and depth to designs, enhancing visual compositions |
| Engineering | Optimizing structural stability, load-bearing capacity, and construction processes |
As seen in the table above, scalene triangles play an integral role in architecture, design, and engineering, making them a valuable mathematical concept within these industries.
Conclusion
In conclusion, the world of scalene triangles reveals fascinating properties that distinguish them from other types of triangles. The unique feature of scalene triangles lies in their non-congruent sides, where each side has a different length. This characteristic results in specific angles within the triangle, contributing to its overall geometric shape.
By understanding the intricacies of scalene triangles, we gain valuable knowledge that can be applied in various fields where geometric shapes play a crucial role. Architecture, design, and engineering are just a few areas that benefit from a deep understanding of the properties and angles of scalene triangles.
As we navigate through the realm of triangles, the scalene triangle stands out as a distinctive geometric shape, offering a wide range of applications. Its asymmetry and non-congruent sides make it a versatile triangle, capable of adapting to different design and mathematical concepts.
FAQ
What is a scalene triangle?
A scalene triangle is a type of triangle that has three different side lengths and three non-congruent sides.
What are the properties of scalene triangles?
Scalene triangles have three different side lengths and angles. The sum of the internal angles of a scalene triangle is always 180 degrees.
How do the angles in a scalene triangle differ from each other?
Unlike an equilateral or isosceles triangle, the angles in a scalene triangle are all different. Each angle can have a unique measure.
How can we calculate the angles in a scalene triangle?
To calculate the angles in a scalene triangle, we can use the law of cosines or the law of sines, depending on the information available about the side lengths and angles.
What are some applications of scalene triangles?
Scalene triangles are used in various fields, such as architecture, engineering, and design. They play a crucial role in determining angles and lengths in structures and shapes.